Area of Parabola Inscribed in a Rectangle

By Jo_Christina752 24 Apr, 2022 Post a Comment

So according to Archimedes the area of the light blue parabolic. Up to 3 cash back A rectangle is inscribed with its base on the x-axis and its upper corners on the parabola y1-x2.

5 What Is The Area Of A Parabola Inscribed In A Rectangle 30 Cm Long And 22 Cm Wide Brainly Ph

The upper vertices being points on the parabola are.

. Formulae of the area of largest rectangle 2ab. Let the upper right corner of the rectangle has co-ordinates x y Then the area of rectangle A 4xy. This answer is not useful.

The negative solution can be ignored since the parabola is symmetric. Area 2 a b. Find the area of the rectangle in terms of x.

Area 2 a b. Show activity on this post. X Af x A x Af x A Switch to the Arrow Tool.

Lets use integral calculus to check the answer we obtained using Archimedes approach. -x9-x2 and x9-x2. A 8 m 2 Area of a parabola inscribed in the rectangle is 8 m 2.

Area 2 10 8. Now we have to maximise A in. 2A circle having an area of 53093 square meters is cut into segments by a chord which is 5 meters from the center of the circle.

Rectangle inscribed in parabola. Use the left-window to plot the following two points. DAdx 4xdydx 4y.

The measure of the base of the rectangle is therefore 2x. My idea is to somehow parametrize rectangle area and then use derivatives to maximize that area but I am not sure if thats correct nor how to do it. A rectangle is inscribed with its base on the x-axis and its upper corners on the parabola y 11 - 2².

Formulae of the area of largest rectangle 2ab. A rectangular area needs to be fenced in such that fencing on one side costs 15 per meter while the other three cost 10 per meter. You can reshape the rectangle by dragging the blue point at its lower-right corner.

Area 160 sq. 20 - 5sqrt43² 20 - 5169 20 - 809 1009 Thus the maximum area is 2sqrt431009 2566001196. A rectangle is inscribed inside the parabola y 8 - x2 and above the x-axis as shown below.

Find the area of the region in the first quadrant that is bounded above by y Vi and below by the X-axis and the line y-r-2. This video provides an example of how to find the rectangle with a maximum area bounded by the x-axis and a quadratic functionSite. A rectangle is to be inscribed in a semicircle of radius 2.

A 3 2 x 4m x 3m. A rectangle is inscribed with its base on the x-axis and its upper corners on the parabola y4-x2. The area of a parabola inscribed in a rectangle 3 meters long with diagonal 5 meters is.

Because of the fact that the parabola is symmetric to the y-axis the rectangle must also be symmetric to the y-axis. The parabola is described by the equation y -ax2 b where both a and b are positive. A rectangle is inscribed between the x-axis and a downward-opening parabola as shown above.

I assume that a rectangle is inscribed in another only all four vertices of the inscribed rectangle touch the outer rectangle. So the outer rectangle itself is the largest and there is either no minimum or a degenerate rectangle having area 0 for the min. Differentiating equation of ellipse with respect to x we have.

A 3 2 x base x height. Here a 6 and b 8 22. The area of the rectangle is A h w.

Thinking of the area as a function of x we have. What are the dimensions of such a rectangle with the greatest. This answer is useful.

What are the dimensions of such a rectangle with the greatest possible area. Rectangle is inscribed between the x-axis and the parabola y16-x2 wiht one side along the x-axis as shown below. 2xa2 2yb2dydx 0.

Use the Polygon tool to draw a polygon around the 4 points. That means that the two lower vertices are -x0 and x0. Compute the area of the smallest segment.

This is a standard calculus demonstration showing the area of a rectangle restricted by a parabola. Area of a parabola is. The height is found by plugging the critical point into the function fx.

Now Equation of ellipse x2a2 y2b2 1. Width - Height A rancher wants to fence in an area of 500000 square feet in a rectangular field and then divide it in half with a fence down the middle parallel to one side. Therefore the measure of the height of the rectangle is.

Find the area of the largest rectangle that can be inscribed in the ellipse x 2 36 y 2 8 1. Using the critical value z sqrt43 the width of the maximum-area rectangle is 2sqrt43. If this was done correctly all 4 points should move when you drag point A.

Here a 10 and b 8. Find the dimensions of the rectangle with the largest area. What is the largest area the rectangie can have and what are its dimensions.

But h depends on w w 2 is the x-distance from the origin w represents width so h a w 2 2. What are the dimensions of such a rectangle with the greatest possible area. I was given a task to maximize the area of a rectangle that can be inscribed between parabola y 1 x 2 and a line y 0 such that one side of the rectangle lies on the x axis.

A w a w 2 4. In my particular example with y x 2 and the line y x 2 intersecting the parabola at 1 1 and 2 4 the pink triangle has width AC 168 units and perpendicular height 402 units so the area is.

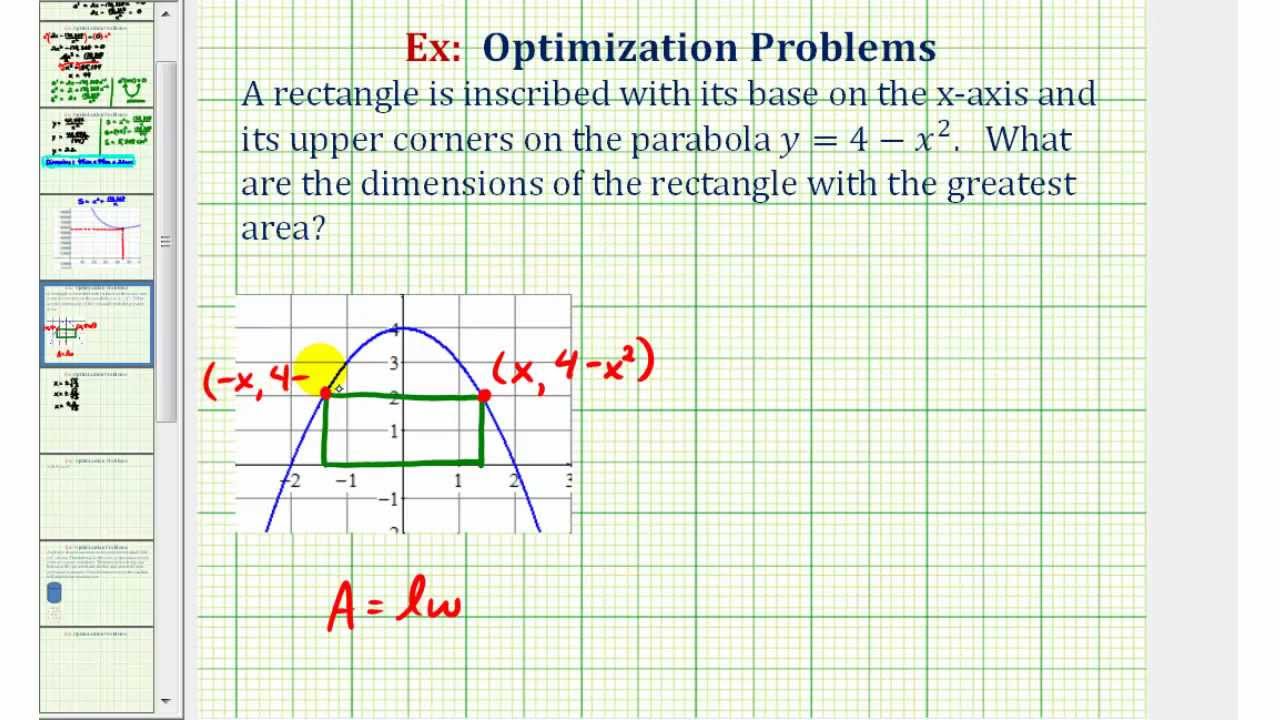
Ex Optimization Maximum Area Of A Rectangle Inscribed By A Parabola Math Help From Arithmetic Through Calculus And Beyond